It's good to have a friend who is patient and knowledgeable when it comes to both martial arts and theoretical physics.
After all, you never know when you might need to know something, like the next move in a kum do form, or the reason the sky reflects blue light. (I got a good technical explanation for that tonight as well, but without the napkin.)
Grateful tonight for his quality coaching, and for Karate Nerd nights in general. We've been having a lot of them since his return from college, and it's been good for our entire group.
Grateful also for some high praise I received tonight from two tough instructors, for a relaxing lunch out with The Master, and for Middle, who finally takes me seriously enough to work on material together in class.
And as always, grateful for Savageman, who juggled sports and childcare and didn't get to come out with us tonight, but who will hopefully join us Thursday. Despite the financial challenges, this chapter in our marriage has shown us certain benefits, including more time to spend together in the company of such wonderful friends.
:-D
*Mass–velocity relationship
In developing special relativity, Einstein found that the kinetic energy of a moving body is
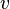 the velocity,
the velocity, 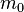 the rest mass, and γ the Lorentz factor.
the rest mass, and γ the Lorentz factor.He included the second term on the right to make sure that for small velocities, the energy would be the same as in classical mechanics:
Einstein found that the total momentum of a moving particle is:

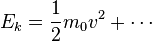
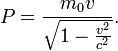
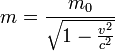

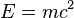
No comments:
Post a Comment